Do you know what is Fibonacci series (Fibonacci and Golden Ratio) is? How is it related to nature? If you also want to read all the important information about the Fibonacci series (Fibonacci and Golden Ratio), then you must read our article on Fibonacci and Golden Ratio in Hindi.
Fibonacci and Golden Ratio
The Fibonacci sequence and the “golden ratio” are two interesting mathematical concepts that have fascinated people for centuries. They are not only of interest to mathematicians but also appear in various forms in nature, art, and architecture.
It is believed that the name Fibonacci sequence was first given to this series by “Édouard Lucas”, a French mathematician, who gave this name in the 19th century. But this Fibonacci sequence has been in use for many years before this. There is a lot of evidence that the first use of the Fibonacci series was in India.
Fibonacci History In India:
In India, too, many mathematicians and some grammarians have worked on the Fibonacci series. Here we have given you detailed information about the history of the Fibonacci series.
Pingala (c. 300-200 BCE)
“The earliest use of this series in India was by Acharya Pingala, an ancient Indian mathematician, in his book Chhandashastra.”
One of the earliest known references to a concept such as the Fibonacci series is found in the work of the ancient Indian mathematician Pingala. In his work on Chhandashastra, Pingala developed a system for calculating the patterns of syllables in Sanskrit poetry.
Although he did not explicitly describe the Fibonacci series, his work on binary numbers and combination patterns of long and short syllables (known as “Maatra Meru”) laid the foundation for what later came to be recognized as the Fibonacci series.
“The Fibonacci series is then used in Bharatanatyam written by Bharat Muni.”
Virahanka (c. 600 AD)
“The best description of this series is through Virahanka but his work on this Fibonacci series is no longer available.”
Indian mathematician Virahanka expanded the work of Pingala and clarified the method of constructing the Fibonacci series. He explained how to calculate the number of ways to form several lengths ‘n’ using 1 and 2, which directly correspond to the Fibonacci sequence. His work provided clear rules for generating these numbers, which are essentially Fibonacci numbers.
Gopala (c. 1135 AD) and Hemchandra (c. 1150 AD)
Then this series became famous as the “Hemchandra series” after the name of a Jain muni who lived in Gujarat, known as “Kali Kalsarvagna”.
Gopala and Hemchandra further developed the ideas of their predecessors. Hemchandra is credited with describing the series clearly in his work on prosody.
The Fibonacci series was in use outside India for a long time. Its importance is also shown in Leonardo of Pisa’s book Liber Abbaci. Leonardo of Pisa counted the number of rabbits with the help of this series.
What is Fibonacci
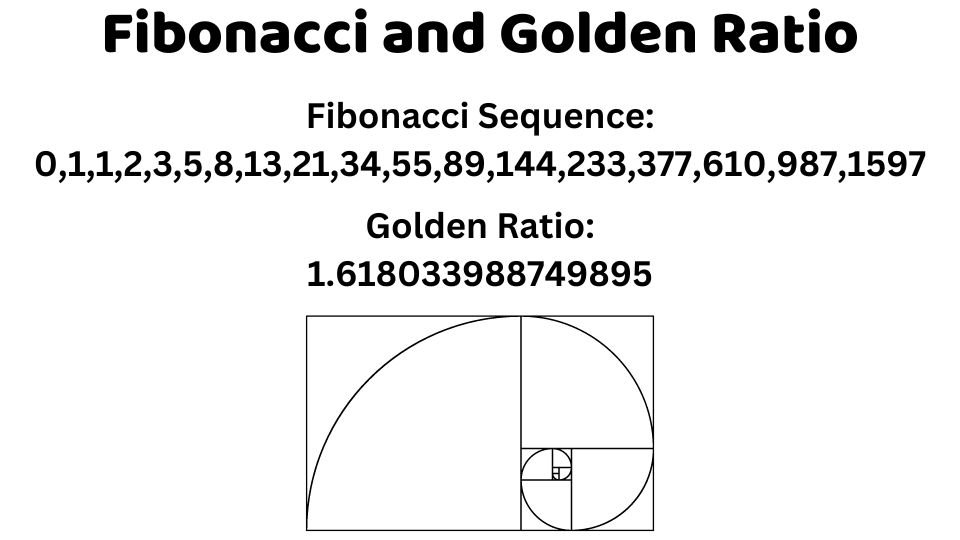
“The Fibonacci sequence is a series of numbers in which each number (after the first two) is the sum of the two preceding numbers. The sequence begins with 0 and 1 and proceeds as follows: 0, 1, 1, 2, 3, 5, 8, 13, 21, 34…….”
This sequence appears in various natural phenomena such as the arrangement of leaves on a stem, the branching of trees, the flowering of artichokes, and the arrangement of bracts of a pine cone.
Mathematics of Fibonacci sequence
The Fibonacci sequence is very easy to create, to find the next number in it, one has to add the previous two numbers in sequence. For example,
Fibonacci Sequence: 0,1,1,2,3,5,8,13,21,34,55,89,144,233,377,610,987,1597……
The first number is 0 and the second number is 1. Now if we want a third number then we have to add the first and second numbers like,
Third number = First number + Second number = 0+1=1
Fourth number = Second number + Third number = 1+1 = 2
Fifth number = Third number + Fourth number =2+1=3
Sixth number = Fourth number + Fifth number = 2+3=5
Seventh number = Fifth number + Sixth number = 3+5=8……..
Golden Ratio
The Golden Ratio, often represented by the Greek letter φ (phi), is approximately equal to 1.618033988749895. It is obtained by dividing two successive terms of the Fibonacci series.
“The two Fibonacci numbers are 5 and 8 respectively, so if 8 is divided by 5, the answer is somewhere around 1.6, which is the Golden Ratio.“
As you move higher in the sequence, the ratio of successive Fibonacci numbers gets closer to the Golden Ratio.

The Golden Ratio has been used in art, architecture, and design to create aesthetically pleasing compositions. It is said to be found in the Parthenon in Greece, the pyramids in Egypt, and even the works of Leonardo da Vinci.
Interesting Fact about “Fibonacci and Golden Ratio”

Fact about Fibonacci
- Fibonacci is also called Nature’s Code. The Fibonacci sequence appears often in nature. For example, the number of petals in many flowers follows a Fibonacci number. Lilies have three petals, buttercups have five, and daisies can have 34, 55, or even 89 petals.
- The arrangement of leaves, seeds, and other plant parts often follows the Fibonacci sequence. In pinecones and pineapples, the spirals of seeds follow the Fibonacci numbers.
- The Fibonacci sequence can be seen in the reproductive patterns of bees. For example, a male bee (drone) has one parent (a mother), while a female bee (worker or queen) has two parents (a mother and a father). The number of ancestors in each generation of a male bee’s family tree follows the Fibonacci sequence.
- The ratio of successive Fibonacci numbers is equal to the Golden Ratio (1.6180339887…). As you move forward in the sequence, the ratio of each pair of successive numbers gets closer to the Golden Ratio.
- Fibonacci numbers are used in computational algorithms and can be found in data structures such as Fibonacci heaps.
Fact About Golden Ratio

- The Golden Ratio has been used for centuries in art and architecture to create visually pleasing compositions. The proportions of the Parthenon in Greece, Leonardo da Vinci’s “Vitruvian Man” and the Egyptian pyramids are often cited as examples of the Golden Ratio.
- The Golden Ratio is used in modern design principles, from logo design (such as the Apple logo) to layout compositions in graphic design and web design. It is believed to create harmony and balance.
- The Golden Ratio is used to predict price movements in the financial markets. Fibonacci retracement levels, which are based on the Golden Ratio, help traders identify potential reversals in price trends.
- The Golden Ratio is seen in the spirals of seashells, hurricanes and the spirals of galaxies. For example, the nautilus shell grows in a logarithmic spiral, where the ratio of the diameter of each turn to the next is approximately the Golden Ratio.
- The human body appears to have proportions that are close to the Golden Ratio. For example, the ratio of hand to forearm length is close to the Golden Ratio, as is the distance from navel to foot and the ratio of body height.
Read It In Other Language: “Hindi“

